Professor Roger H. Rangel
Ph.D. Student: Saad Alabduljalil
This work presents the inviscid temporal stability analysis of an unbounded shear layer of two fluids of different viscosity and density. Two background velocity profiles are considered: the piecewise-linear profile and the more realistic error-function profile. A detailed study for the disturbance kinetic energy is carried out to physically understand the mechanism that causes instability to occur. The surface tension effect is investigated extensively. Surface tension is found to destabilize the neutrally-stable waves that present in W=0 case. This new unstable mode becomes more independent from the other stronger mode and extremely less evident when the viscosity and/or density difference increases. It is shown that short waves are destabilized by a small amount of surface tension. This behavior can be attributed to the discontinuity in the tangential velocity at the interface caused by surface tension. Also, surface tension might slightly shift the phase difference of the perturbation velocities outside the stable quadrants, which is analogous to the Prandtl’s (1935) theory for viscosity diffusion. When surface tension is sufficiently large to overcome the destabilizing effect of the Reynolds stress, it starts to stabilize all wavelengths. Short-wavelength instability is observed with a background viscosity jump at the interface. A comparison between the two velocity profiles is presented. The piecewise-linear profile fails to provide acceptable results for short waves especially in nonhomogeneous shear-layer flows; however, the phase speed results are in a good agreement with those of the error-function profile.
References
- Drazin, P. G. & Howard, L. N. 1962 The instability to long waves of unbounded parallel inviscid flow. J. Fluid Mech. 14, 257-283.
- Drazin, P. G. & Reid, W. H. 1981 Hydrodynamic Stability. Cambridge University Press.
- Esch, R. E. 1957 The instability of a shear layer between two parallel streams. J. Fluid Mech. 3, 289-303.
- Garcia, R. V. 1956 Barotropic waves in straight parallel flow with curved velocity profile. Tellus 8, 82-93.
- Huerre, P. & Rossi, M. 1998 Hydrodynamic instabilities in open flows. In Hydrodynamics and Nonlinear Instabilities (ed. C.Godrèche & P. Manneville), pp. 81-294. Cambridge University Press.
- Lindsay, K. A. 1984 The Kelvin-Helmholtz instability for a viscous interface. Acta Mechanica 52, 51-61.
- Mathieu, J. & Scott, J. 2000 An Introduction to Turbulent Flow. Cambridge University press.
- Michalke, A. 1964 On the inviscid instability of the hyperbolic-tangent velocity profile. J. Fluid Mech. 19, 543-556.
- Michalke, A. 1972 The instability of free shear layers. In Progress in Aerospace Sciences (ed. D. Kuchemann et al.), pp. 213-239. Pergamon Press.
- Miles, J. W. 1959 On the generation of surface waves by shear flows. Part 3. J. Fluid Mech. 7, 583-598.
- Prandtl, L. 1935 The mechanics of viscous fluids. In Aerodynamic Theory (ed. W. F. Durand), pp. 34-208. J. Springer.
- Rangel, R. H. & Sirignano, W. A. 1988 Nonlinear growth of Kelvin-Helmholtz instability: Effect of surface tension and density ratio. Physics of Fluids 31, 1845-1855.
- Rosenhead, L. 1963 Laminar Boundary Layers. Clarendon Press.
- Tatsumi, T. & Gotoh, K. 1960 The stability of free boundary layers between two uniform streams. J. Fluid Mech. 7, 433-441.
- Tatsumi, T., Gotoh, K. & Ayukawa, K. 1964 The stability of a free boundary layer at large Reynolds numbers. J. Phys. Soc. Jpn. 19, 1966-1980.
- White, F. M. 1991 Viscous Fluid Flow, 2nd Edn. McGraw-Hill.
- Yih, C.-S. 1967 Instability due to viscosity stratification. J. Fluid Mech. 27, 337-352.
- Zalosh, R. G. 1976 Discretized simulation of vortex sheet evolution with buoyancy and surface tension effects. AIAA Journal 14, 1517-1523.
Figures
Fig. 1: Dimensionless disturbance growth rate versus the dimensionless wave number for a homogeneous shear layer with different surface tension values. (a) Piecewise-linear profile, (b) error-function profile.
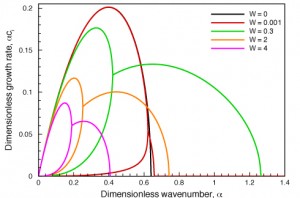
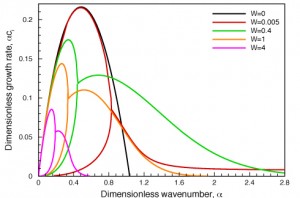
Fig. 2: Temporal variation of the dimensionless disturbance kinetic energy versus the dimensionless wavenumber for a homogeneous shear layer at different surface tension values. (a) Piecewise-linear profile, (b) error-function profile.
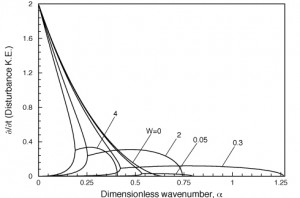

Fig. 3: Dimensionless disturbance growth rate versus the dimensionless wavenumber for a homogeneous piecewise-linear profile with different surface tension values: the second unstable region. This behavior, i.e. discrete unstable wavenumber spectrum, is not seen in the error-function profile.
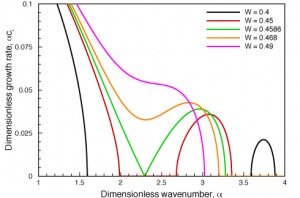
Fig. 4: Comparison between the dimensionless growth rate of the piecewise-linear profile and the error-function profile for a homogeneous shear-layer flow. (a) W=0.4, (b) W=0.
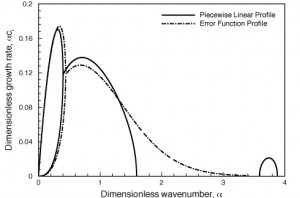
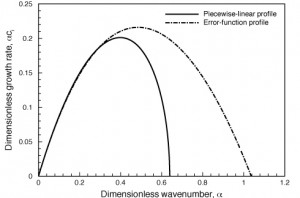
Fig. 5: Comparison between the dimensionless phase speed of the piecewise-linear profile (dash line) and the error-function profile (solid line) for a homogeneous shear-layer flow with W=0.005 (thick line) and 1 (thin line).
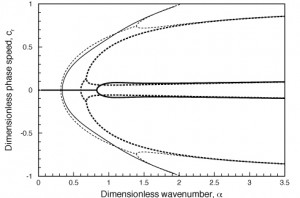
Fig. 6: Dimensionless disturbance growth rate versus the dimensionless wavenumber for a same-density piecewise-linear profile at various viscosity ratios and W=5. (a) m<1, (b) m>1.
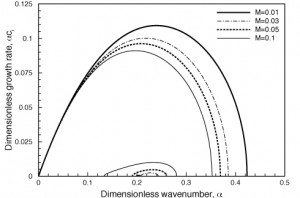
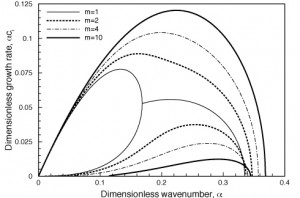
Fig. 7: Comparison between the dimensionless growth rate of the piecewise-linear profile and the error-function profile for a same-density shear-layer flow at different viscosity ratios greater than one and W=0.
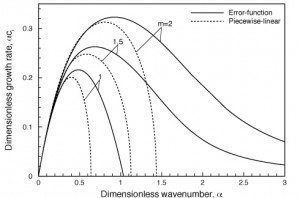
Fig. 8: Dimensionless disturbance growth rate versus the dimensionless wavenumber for a piecewise-linear profile at different W values. (a) s=m=0.9, (b) s=m=0.3.
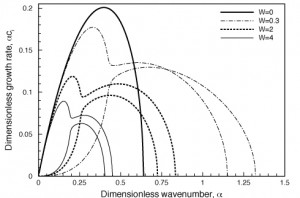
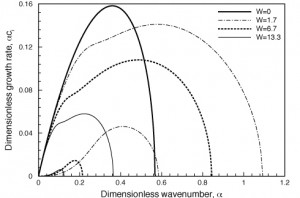
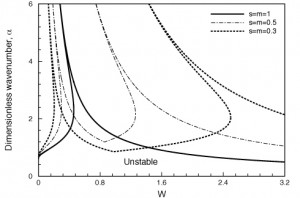
Fig. 9: Stability boundary for a piecewise-linear profile at different s and m.