Professor Roger H. Rangel
Jean-Pierre Delplanque
An Improved Model for Droplet Solidification on a Flat Surface
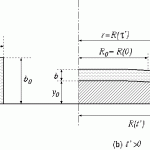
An existing model of the deformation and solidification of a single droplet impinging on a cold surface (Fig. 1) has been revised and improved. The original model (Fig. 2) is based on a two dimensional axisymmetric flow approximation of the velocity field, the Neumann solution to the one-dimensional Stefan solidification problem, and an integral mechanical energy balance. The improved model features a more appropriate velocity field which satisfies the no-shear boundary condition at the free surface, and an accurate derivation of the dissipation term from the mechanical energy equation. This equation is solved numerically (Fig. 3).
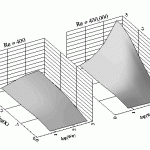
Comparisons of the original and the improved models are performed. Results show that the original model over-estimates the final splat size by about 10%. The discrepancy is more pronounced at larger Weber numbers, where viscous effects dominate (Fig. 4).
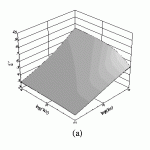
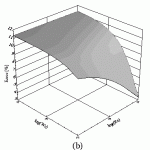
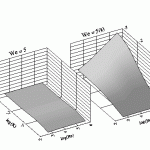
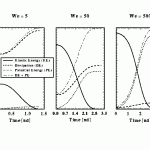
The effects of the Weber number, the Reynolds numbers, and the solidification parameter are investigated through detailed numerical calculations (Figs. 5 and 6). Two regimes of spreading/solidification have been identified. If Re/We is small, the process is one of dissipation of the incident droplet kinetic energy; whereas for large values of Re/We the process can rather be characterized as a transfer between kinetic and potential energy (Fig. 7). In the latter case, the variations of the final splat size versus the solidification constant exhibit a non-monotonic behavior. This indicates that, for a given material, the deposition process can be optimized. Correlations relating the final splat size to the process parameters are given.
Selected Figures

Downloadable Publications
- Delplanque, J.-P. and Rangel, R.H., “An Improved Model for Droplet Solidification on a Flat Surface” , Submitted for publication in The Journal of Materials Science, 1996.